Experimental Designs: Factorial Designs

Classical design such as fractional factorial designs and response surface designs, are standard designs with set numbers of runs for a set number of parameters.

Factorial designs (2-level design) can be either:

Full Factorial: all combination of factors at each level. With k factors at 2 levels – 2^k experiments
Fractional Factorial: a balanced fraction of the full factorial i.e. doing fewer experiments while still gaining maximum information.

However, there is a penalty by reducing the resource i.e. increasing the amount of aliasing. Aliasing occurs when there is not enough experiments to fully estimate all the potential terms of a model. This creates confounding variables which is a factor whose presence affects the factor(s) being studied. This means that the results you get do not reflect the actual relationship between the factor(s) being studied and the response. The degree of aliasing changes the resolution of a design: it is dependent on the number of parameters studied and the number of runs as shown in Table 1.

Table 1
# runs	2 factors	3 factors	4 factors	5 factors	6 factors	7 factors	8 factors	9 factors
4	Full	1/2
8		Full	1/2	1/4	1/8	1/16
16			Full	1/2	1/4	1/8	1/16	1/32
32				Full	1/2	1/4	1/8	1/16
64					Full	1/2	1/4	1/8
128						Full	1/2	1/4

Resolution V design (Green): models main terms and all 2-factors interactions

Resolution IV design (Blue): main terms can be identified but 2-factors interactions confounded with other 2-factors interactions

Resolution III design (Red): main effects are aliased with 2-factor interactions so there is a risk to miss potential interactions.

Figure 1: Resolution III fractional factorial design for 3 factors in 4 runs + 2 centre points — **Figure 1:** Resolution III fractional factorial design for 3 factors in 4 runs + 2 centre points

The higher the degree of aliasing, the harder it is to interpret the design and more work may be needed to reduce aliasing. However, a classical design (Figure 1) has the ability to be augmented by doing a foldover design (Figure 2) which gives the next highest resolution design with less aliasing.

Centre Points are experiments run at the mid point of each factor range. They allow to check for curvature (see later). Replicates provide you with the ability to compute estimates of pure error. Centre points are often run in replicates.

Figure 2: Foldover design include other extreme and 2 additional centre points which give the full fractional design — **Figure 2:** Foldover design include other extreme and 2 additional centre points which give the full fractional design

Fractional factorial designs are traditionally used to identify key parameters controlling a response and the presence of any interactions. Here, the focus is on initial process understanding to move towards a set of optimal operating conditions that meet pre-defined criteria (eg. high purity and high yield). When large number of parameters are being investigated, other types of design can be more efficient.

Figure 3 is an example of output from statistical analysis (using Design-Expert®). A process was studied by varying three parameters:

A: time, B: temperature and C: concentration

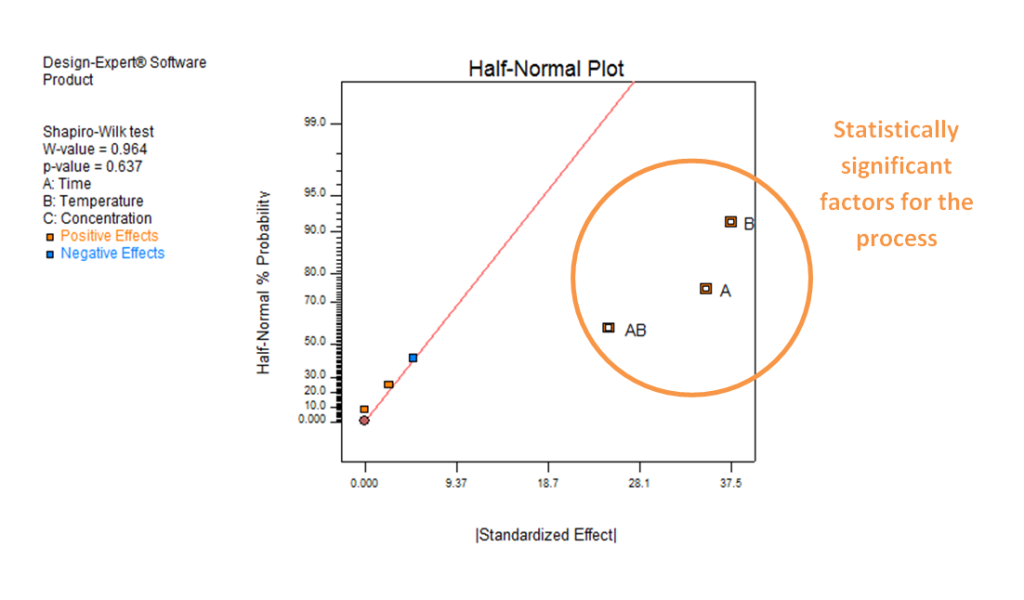
Figure 3: An example of output from statistical analysis (using Design-Expert®) — **Figure 3:** An example of output from statistical analysis (using Design-Expert®)

The half-normal plot for the product formation shows that two of those factors statistically significant for the formation of the product, time and temperature as well as their interaction.

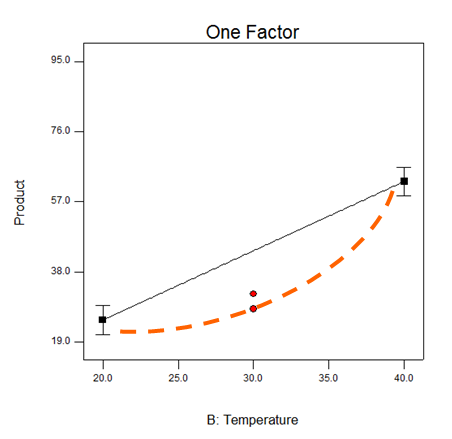
Figure 4: Graph demonstrating curvature: centres points do not fit the predicted linear model — **Figure 4:** Graph demonstrating curvature: centres points do not fit the predicted linear model

Classical screening designs are linear and always model a straight line between two points. However, this is not always the case for the real system and non-linear effects can be detected through the use of replicates at the centre (Figure 4). This phenomenon is called curvature and a different type of design is needed to more accurately model this effect.